Scientists Say Pure Chance Could Account For German Ace's
Tally
by Aero-News Senior Correspondent Kevin R.C. "Hognose"
O'Brien
 The Red Baron, mystery
man and tragic figure of the First World War, has inspired novels,
multiple biographies, an autobiography, innumerable magazine
articles, about 2,890,000 Google hits and a pizza company. Really.
He's also loomed large over several feature films, including The
Blue Max (1966) and the forthcoming Flyboys, without himself
becoming a central character in them.
The Red Baron, mystery
man and tragic figure of the First World War, has inspired novels,
multiple biographies, an autobiography, innumerable magazine
articles, about 2,890,000 Google hits and a pizza company. Really.
He's also loomed large over several feature films, including The
Blue Max (1966) and the forthcoming Flyboys, without himself
becoming a central character in them.
But now he's appeared in an entirely new venue: an academic
paper. The paper, written by Drs. Mikhail
Simkin and Vwani P. Roychowdhury of UCLA's
Electrical Engineering department, is called "Theory of Aces: high
score by skill or luck?" The paper advances the controversial idea
that Manfred Freiherr von Richthofen wasn't all that special; and
that while he was a better than average fighter pilot, luck
accounted for most if not all of his success.
The paper has been released on the internet but not
published.
Simkin and Roychowdhury previously studied a theory that chance
played a larger role than merit in the fame of fighter pilots; that paper was published in the
Journal of Mathematical Sociology earlier this year. But while the
first paper was mildly controversial with Red Baron fans, the new
one is tantamount to dropping an insulting note tied to a spanner,
right smack dab into the middle of their aerodrome.
"[T]he probability to achieve by pure chance Manfred von
Richthofen's 80 victories is one, divided by to the 80th power or
10 to the -21 power. One is tempted to conclude that high-scoring
aces had outstanding skills. A more careful analysis proves this
conclusion wrong."
It's a bold statement. Simkin (himself a private pilot) and
Roychowdhury conclude, in fact, that Richthofen's run of 80
victories might have been pure chance... or to put it in a
non-scientific way, dumb luck.

"Let us, given the ratio of the number of defeats to the number
of victories, compute the probability to get Richthofen's score.
Assuming that the ratio of the probability of credited victory to
the probability of defeat is 8.2, the probability of defeat in each
decisive engagement is "r"= 1/9.2 or approximately equal to 0.11.
The probability of 80 victories in a row is (1-"r") to the 80th
power, which resolves to 0.89 to the 80th, or approximately 10 to
the -4 power. The probability that at least one of 2890 German
fighter pilots will achieve 80 or more victories... is
approximately equal to 0.25. Richthofen's score is thus within the
reach of chance."
From there, they go on to calculate the distribution of
victories, given the "r" of 0.11 that they previously derived, and
conclude that, "it is clear that chance can account for most of the
variance in the numbers of victories."
Simkin and Roychowdhury still aren't done. They went on to
calculate what they called "intrinsic defeat rates." The IDR is the
likelihood for individual pilots of defeat or victory... in other
words, skill at air combat. It changes over time, both as the
pilots who are less skilled are killed, captured, or sidelined by
wounds, and as the survivors' skills increase.
The math gets harder to follow here (and a lot harder to
represent in HTML or by words) so if you want to see the sheet
music, download the original paper [Note: .pdf!] We'll
skip right to the conclusion (and show the graph):
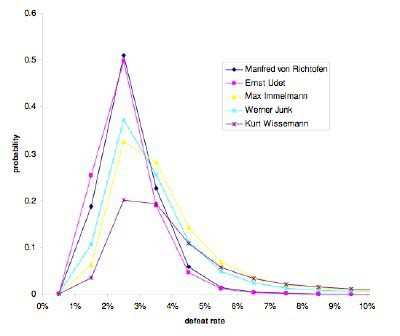
"The inference for five representative pilots,... is given in
[the graph]. In particular, Manfred von Richthofen most likely had
the intrinsic defeat rate of 2.5%. According to the distribution of
intrinsic defeat rates... about 29% of pilots have [a] defeat rate
of 2.5% or lower. This means that MvR is most likely merely in top
29% according to his skill."
While all one's flying instinct cries foul at this, it's hard to
argue with the numbers. True, chance is always a factor; many
fighter pilots never see an enemy or fire a shot in their careers,
even in wartime. The pilots of non-fighter aircraft figure in this
study mostly as targets; it is probable that some pilots of higher
potential ability were assigned to other kinds of flying where they
had no hope of aerial victories. Able pilots might have been undone
by balky engines or jamming guns; pilots of lesser natural ability
might have tried to even the odds by, for instance, by carefully
measuring and lubricating his ammunition. In the end it's scarcely
possible to truly separate the influence of chance and skill. But
the authors have been quite daring to try.
Richthofen himself clearly felt that chance was a factor in his
life and death. Not long before his demise, he wrote this:
"[D]death may be right on my neck and I often think about it.
Higher authority has suggested that I should quit flying before it
catches up with me." He resolved not to quit, because he'd be
ashamed to take advantage of his fame, when the average German
soldier in the trenches had no such way out.

The authors point out that while others have suggested that
people perceived as remarkably skillful may merely have been lucky,
they are the first ones to tackle this argument with real data,
taking both their previous paper and this one together. For this
argument to be accepted scientifically, their results have to be
repeated.
Anyone have a copy of Mathematica and a good data set on another
group of aces?
 NTSB Final Report: Cozy Cub
NTSB Final Report: Cozy Cub ANN FAQ: Contributing To Aero-TV
ANN FAQ: Contributing To Aero-TV Classic Aero-TV: Seated On The Edge Of Forever -- A PPC's Bird's Eye View
Classic Aero-TV: Seated On The Edge Of Forever -- A PPC's Bird's Eye View ANN's Daily Aero-Linx (04.29.25)
ANN's Daily Aero-Linx (04.29.25) ANN's Daily Aero-Term (04.29.25): Execute Missed Approach
ANN's Daily Aero-Term (04.29.25): Execute Missed Approach






